The Angle Pair Relationships with Parallel Lines Worksheet Answer Key provides a comprehensive guide to understanding the relationship between angle pairs and parallel lines. This essential resource offers clear definitions, engaging examples, and practical practice problems to help students master this fundamental geometry concept.
Delving into the intricacies of angle pair relationships, this worksheet explores the four main types: complementary, supplementary, vertical, and adjacent. Each type is thoroughly explained with illustrative examples, allowing students to grasp the unique properties and characteristics that distinguish them.
Angle Pair Relationships with Parallel Lines: Angle Pair Relationships With Parallel Lines Worksheet Answer Key
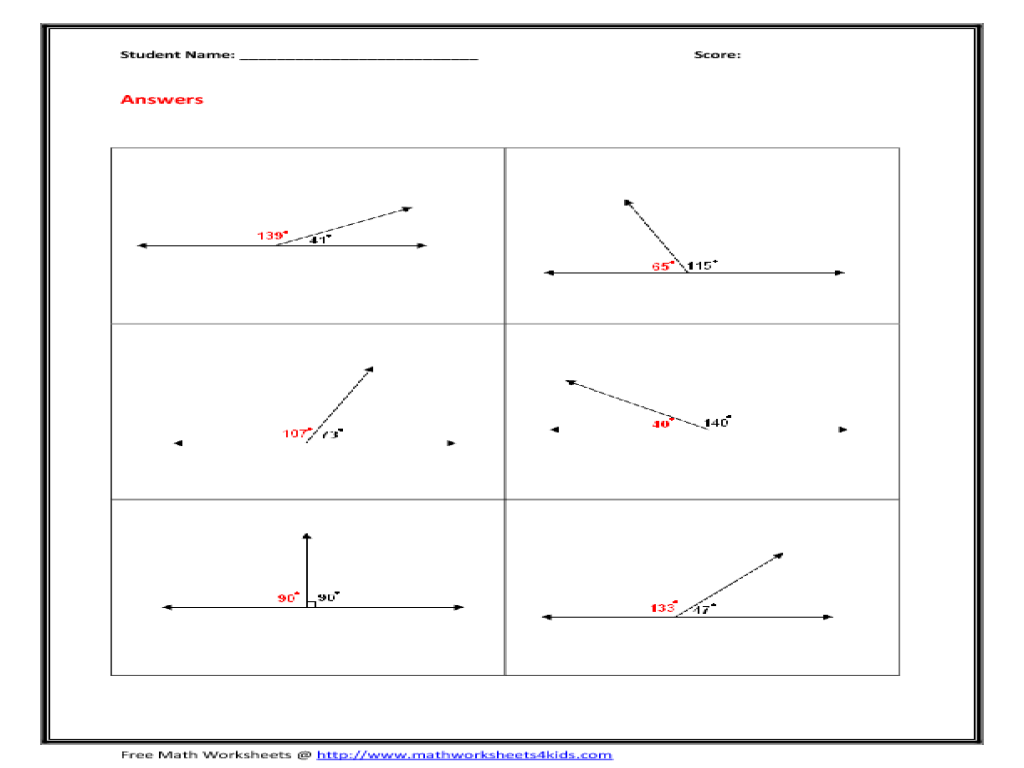
Angle pair relationships are geometric relationships between pairs of angles formed by intersecting lines. These relationships can be used to determine whether lines are parallel.
Definitions and Concepts, Angle pair relationships with parallel lines worksheet answer key
Angle pair relationships:Geometric relationships between pairs of angles formed by intersecting lines.
Parallel lines:Lines that never intersect.
Relationship between angle pair relationships and parallel lines:Certain angle pair relationships indicate that lines are parallel.
Angle Pair Relationships
- Complementary angles:Two angles whose sum is 90 degrees.
- Supplementary angles:Two angles whose sum is 180 degrees.
- Vertical angles:Two opposite angles formed by intersecting lines.
- Adjacent angles:Two angles that share a common vertex and a common side.
Parallel Lines and Angle Pair Relationships
Certain angle pair relationships indicate that lines are parallel:
- If two lines are intersected by a transversal and the alternate interior angles are congruent, then the lines are parallel.
- If two lines are intersected by a transversal and the same-side interior angles are supplementary, then the lines are parallel.
Limitations:Angle pair relationships can only be used to determine if lines are parallel if the transversal intersects the lines in distinct points.
Practice Problems
| Angle Pair Relationship | Condition for Parallel Lines |
|---|---|
| Alternate interior angles | Congruent |
| Same-side interior angles | Supplementary |
Answer key:
- If the alternate interior angles are congruent, then the lines are parallel.
- If the same-side interior angles are supplementary, then the lines are parallel.
User Queries
What are the four types of angle pair relationships?
Complementary, supplementary, vertical, and adjacent.
How can angle pair relationships be used to determine if lines are parallel?
If two lines intersect to form congruent vertical angles, then the lines are parallel.
What are the limitations of using angle pair relationships to determine if lines are parallel?
Angle pair relationships can only be used to determine if lines are parallel if the lines intersect.