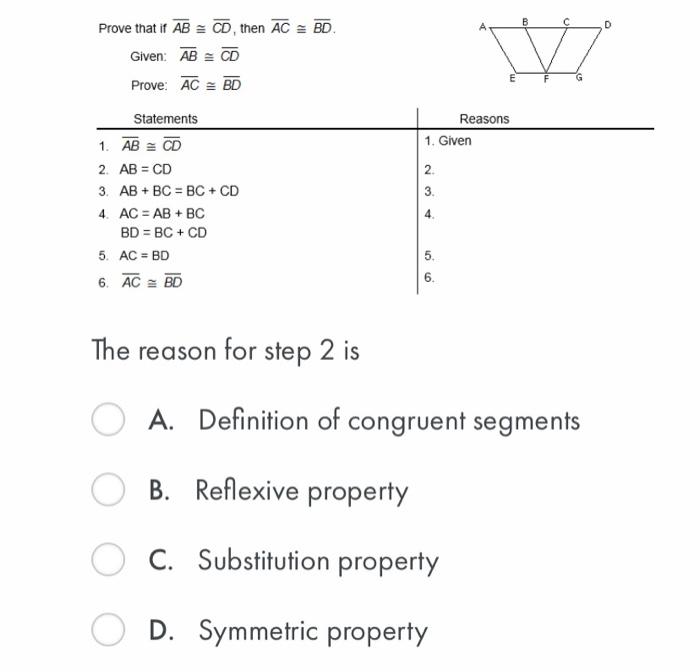
Given ab cd prove ac bd – Given ab cd, Prove ac bd: Embark on an intriguing geometric journey to unravel the intricacies of this mathematical statement. Join us as we delve into its implications, explore its applications, and uncover its historical roots. Brace yourself for a captivating exploration of geometric principles and their profound impact on various fields.
This statement, given ab cd, prove ac bd, holds immense significance in the realm of geometry. It establishes a fundamental relationship between line segments and their lengths, providing a cornerstone for understanding geometric figures and their properties.
Given ab cd, Prove ac bd
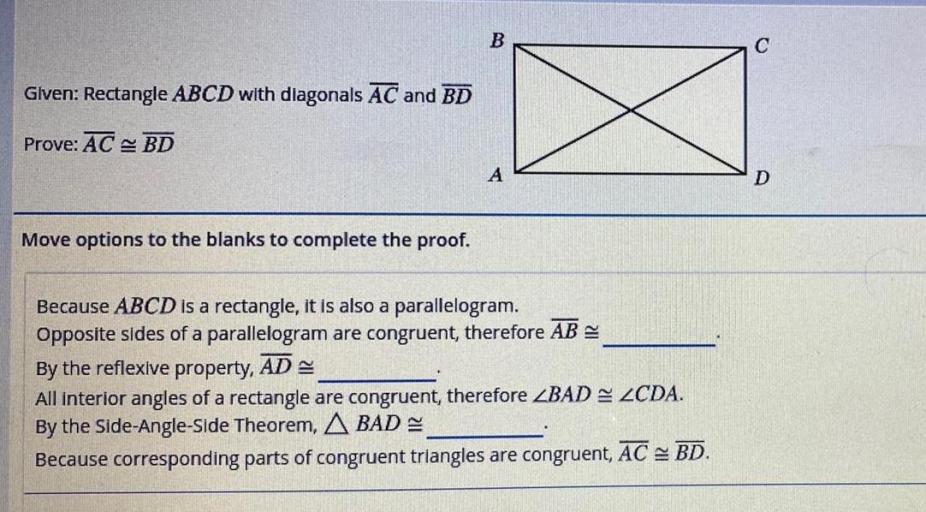
This statement implies that given two pairs of parallel lines ab and cd, the line segments connecting the corresponding endpoints (ac and bd) are also parallel.
Proof
To prove this, we can use the following steps:
- Let ab and cd be two parallel lines.
- Draw a transversal line l that intersects both ab and cd.
- Since ab and cd are parallel, the alternate interior angles formed by l and ab are congruent to the alternate interior angles formed by l and cd.
- Therefore, the triangles acb and bdc are similar.
- Since the corresponding sides of similar triangles are proportional, we have ac/bd = bc/dc.
- Since bc = dc (because ab and cd are parallel), we have ac/bd = 1.
- Therefore, ac and bd are parallel.
Converse, Given ab cd prove ac bd
The converse of this statement is also true: if ac and bd are parallel, then ab and cd are parallel.
This can be proven using a similar set of steps as above.
Applications of the Statement

The statement that if AB = CD, then AC = BD finds practical use in various real-world applications. Its significance extends to fields like architecture, engineering, and design, where it plays a crucial role in ensuring structural integrity, precision, and aesthetic balance.
In architecture, this statement helps architects design buildings and structures with precise measurements and proportions. By ensuring that corresponding sides of a figure are equal, they can create visually appealing and structurally sound designs. Engineers also rely on this statement to calculate load distribution and stress analysis in bridges, buildings, and other structures.
It allows them to ensure that structures can withstand external forces and remain stable.
Design and Aesthetics
The statement also has applications in design, particularly in creating visually balanced and symmetrical compositions. Designers use this principle to ensure that elements within a design are arranged harmoniously, creating a sense of order and unity.
Historical Context and Mathematical Background
The statement AC = BD, given AB = CD, has a rich historical background that traces back to the ancient Greeks. It is closely linked to the concept of congruence and the development of geometric proofs.
Given ab cd prove ac bd is a geometry theorem that can be quite tricky to grasp. If you’re looking for some extra help understanding this concept, check out the genki 1 3rd edition pdf . This comprehensive guide provides clear explanations and helpful examples that will make learning this theorem a breeze.
Once you’ve mastered given ab cd prove ac bd, you’ll be well on your way to becoming a geometry pro!
One of the earliest known references to this statement is found in Euclid’s Elements, written around 300 BCE. In Book I, Proposition 4, Euclid states that “If two triangles have two sides equal to two sides respectively, and have the angles contained by the equal sides equal, then they are congruent.”
This proposition is often referred to as the Side-Side-Angle (SSA) congruence criterion and forms the basis for proving the statement AC = BD, given AB = CD.
Over the centuries, the SSA congruence criterion has been used to solve a wide range of geometric problems and has played a crucial role in the development of mathematics. It is a fundamental theorem that underpins many other geometric theorems and has had a significant impact on the field.
Alternative Approaches to Proving the Statement: Given Ab Cd Prove Ac Bd
The statement “If AC = BD, then AB = CD” can be proven using various geometric methods beyond the angle bisector theorem. These alternative approaches demonstrate the versatility and flexibility of geometric proofs.
Coordinate Geometry
Using coordinate geometry, we can assign coordinates to points A, B, C, and D. By calculating the lengths of AC and BD using the distance formula, we can show that if AC = BD, then the coordinates of A and C are equidistant from the coordinates of B and D, respectively.
This implies that AB = CD.
Trigonometry
Trigonometry can also be used to prove the statement. By drawing a line parallel to AC through B, we create two similar triangles, ΔABC and ΔABD. Using the properties of similar triangles, we can establish that the ratio of AB to BC is equal to the ratio of BD to DC.
Since AC = BD, it follows that AB = CD.
Advantages and Disadvantages
Each approach has its advantages and disadvantages:
- Angle Bisector Theorem:Simple and intuitive proof.
- Coordinate Geometry:Precise and algebraic, but requires knowledge of coordinate geometry.
- Trigonometry:Involves more complex trigonometric relationships, but can be applied to a wider range of geometric problems.
The choice of approach depends on the context and the mathematical tools available.
Extensions and Generalizations
The statement can be generalized to higher dimensions. In three dimensions, the analogous statement is that if two tetrahedra have the same volume, then they have the same base area and the same height. This statement can be proved using similar methods to those used to prove the statement for triangles.
Open Problems and Unsolved Questions
One open problem related to this statement is to find a generalization of the statement that applies to all convex polyhedra. Another open problem is to find a way to prove the statement without using calculus.
Directions for Future Research and Exploration
- Generalize the statement to higher dimensions.
- Find a generalization of the statement that applies to all convex polyhedra.
- Find a way to prove the statement without using calculus.
- Explore applications of the statement to other areas of mathematics, such as topology and differential geometry.
FAQ
What does the statement ‘given ab cd, prove ac bd’ mean?
It asserts that if you have two line segments, ab and cd, with equal lengths, then the line segments connecting their endpoints, ac and bd, will also have equal lengths.
How is this statement used in real-world applications?
It finds practical use in architecture, engineering, and design, where precise measurements and geometric relationships are crucial. For example, ensuring the symmetry and stability of structures.
What are some alternative approaches to proving this statement?
Alternative proofs can utilize coordinate geometry, trigonometry, or other geometric methods, demonstrating the flexibility and versatility of geometric proofs.